A hexagon shader in OSL, second edition

A while ago I made a simple OSL shader for hexagonal tilings. Prompted by some questions on BlenderArtists I decided to create a more versatile version that retained the color options but added the distance to the closest edge. That feature may help in creating crips edge patterns because the previously availble distance to the closest center creates rounded shapes that might be suitable for bee hives but not for hard edged stuff like floor tiles etc.

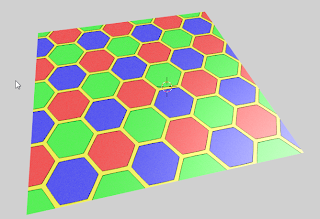
The noodle used to create the image is shown below (click to enlarge):

The color code has stayed the same except for the calculation of the distance to the edge. This might be a bit inefficient, but at least it's easy to read.
The additions are shown below, the full code is available on GitHub.

    // distance to nearest edge
    
    float x = mod(Coordinates[0]/3,1.0);
    float y = mod(Coordinates[1]/3,A2);
   
    #define N 18
    vector hc[N] = {
        vector(  0, -A2/3     ,0),
        vector(  0,     0     ,0),
        vector(  0,  A2/3     ,0),
        vector(  0,2*A2/3     ,0),
        vector(  0,  A2       ,0),
        vector(  0,4*A2/3     ,0),

        vector(0.5, -A2/3+A2/6,0),
        vector(0.5,     0+A2/6,0),
        vector(0.5,  A2/3+A2/6,0),
        vector(0.5,2*A2/3+A2/6,0),
        vector(0.5,  A2  +A2/6,0),
        vector(0.5,4*A2/3+A2/6,0),

        vector(1.0, -A2/3     ,0),
        vector(1.0,     0     ,0),
        vector(1.0,  A2/3     ,0),
        vector(1.0,2*A2/3     ,0),
        vector(1.0,  A2       ,0),
        vector(1.0,4*A2/3     ,0)
    };
    
    float d[N], t;
    
    for(int i=0; i < N; i++){
        float dx = x - hc[i][0];
        float dy = y - hc[i][1];
        d[i] = hypot(dx, dy); 
    }
    
    for(int j= N-1; j >= 0; j--){
        for(int i= 0; i < j; i++){
            if(d[i] > d[i+1]){ 
                SWAP(t, d[i], d[i+1]);
            }
        }
    }
    
    Center  = d[0];
    Edge    = d[1] - d[0];
    InEdge  = Edge < Width;

The approach we have taken is very simple: the hc enumerates all nearby hexagon centers. We then calculate all the distances to these points and sort them shortest to longest (yes with a bubble sort: with 18 elements it might just be faster to do it with a more efficient sorting algorithm at the cost of much more complex code so I don't bother).
The Edge is not realy the distance to the closest edge but the difference between the closest center and the next closest. Near the edge these values are more and more the same so Edge will approach zero. For convience we provide a comparison with some threshold also.

If you would like to know more about programming OSL you might be interested in my book "Open Shading Language for Blender". More on the availability of this book and a sample can be found on this page.

Inheritance and mixin classes vs. Blender operators

recently I was working on an addon that would offer functionality both in weight paint mode and in vertex paint mode. There were slight differences in functionality of course so I needed two operators but I wanted to keep most of the shared code in a single mixin class.

Sharing member functions was easy enough using a mixin class but for some reason for properties this didn't work. Any property I put in the mixin class was not accessible from the derived classes. What was happening here?

class mixin(bpy.types.Operator):
  foo = FloatProperty()
  def oink(self): pass

class operator1(mixin):
  def execute(self, context):
    self.oink()  # ok
    bar = self.foo  # error :-(

However, if I didn't derive the mixin class from bpy.types.Operator all was well ...

class mixin:
  foo = FloatProperty()
  def oink(self): pass

class operator1(bpy.types.Operator, mixin):
  def execute(self, context):
    self.oink()  # ok
    bar = self.foo  # ok too :-)

So what is happening here? Sure in the first scenario both the mixin and the derived class inherit from bpy.types.Operator but that shouldn't be a problem is it? after all, Python's inheritance model supports the diamond pattern.

The answer to this riddle is hidden in Blender's source code, quote:

/* the rules for using these base classes are not clear,
 * 'object' is of course not worth looking into and
 * existing subclasses of RNA would cause a lot more dictionary
 * looping then is needed (SomeOperator would scan Operator.__dict__)
 * which is harmless but not at all useful.
 *
 * So only scan base classes which are not subclasses if blender types.
 * This best fits having 'mix-in' classes for operators and render engines.
 */

So upon creating an instance of a class that is derived from bpy.types.Operator only the class itself and any base classes that are not derived from Blender types are searched for properties. This will reduce needless traversal of base classes that do not define properties themselves and avoid duplicate searches in cases where there is a diamond pattern but it sure is confusing.

It does make sense though: instantiation of operators should be as cheap as possible because instantion happens each time a screen area with the operator controls is redrawn! And this can add up: merely moving your mouse can result in tens of instantiations.

So the moral of this story? Sure it's awkward, especially while it's not well documented, but it is an understandable design choice. There is one serious drawback though; if you have the mixin and your operators in the same file you cannot use register_module() because that function tries to register all classes even the mixin class and that will fail.

conclusion

When using inheritance with bpy.types.Operator derived classes and you want to define properties in a mixin class make sure that:

• the mixin class does not derive from bpy.types.Operator,
• the final classes do derive from bpy.types.Operator (and from the mixin of course),
• you don't use register_module() but use register_class()
for each operator separately (but do not register the mixin)

Blender addon: Assign random vertex colors to connected vertices

Prompted by a request in this BlenderArtists thread I created a small add-on that assigns random vertex colors to regions with connected vertices.

The image shows a single object consisting of several cubes. within each cube all vertices are connected and therefore get the same (but random) color.

Code availability

All functionality of this add-on plus a lot more is now available as a convenient all-in-one add-on on BlenderMarket. It comes with an extensive PDF manual and your purchase will encourage me to develop new Blender add-ons.

The code for the functionality shown in this article is available on GitHub. use File -> User preferences ... -> Addons -> Install from file to install it and don't forget to enable the check box. It will then be available in the Paint menu in Vertex Paint mode.

For discussion you might want to check here